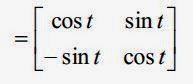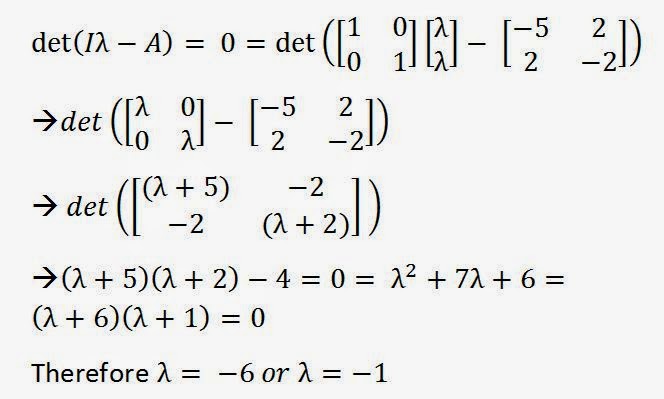Cardboard Boxes, Measure for Measure and Exponential Functions

CARDBOARD BOXES!

We bought some speakers,
my little bro drew my face,
I struggled to breathe,
It was awesome.

 Measure For Measure 

So call back auditions were last night. And WOW, what a fantastic cast we have. The auditions were so much fun as well. Mostly a bunch of theatre sports and some individual monologues. It was a little bit nerves, a little bit because I was surrounded by hilarious people but I was in hysterics most of the night. 10/10 would audition again! I'm so excited to start rehearsals with whatever part I get, even if I only have one line. That is how awesome the cast is! <3

Difficulty Ratings

So I've decided that I'm going to introduce difficulty ratings to different sections in my blog. Though I encourage you to give the harder things a read this means that you can just cruise through the easier parts if you want :D
This is the system I will be using:

5 - ((I Don't Even)): This means it's beyond me and my understanding. There probably won't be much of this because if I don't understand something I will probably not write it so that you all still think I'm smart.

4 - ((Break a Sweat)): This means that I found it difficult but got there in the end

3 - ((So-So): It was relatively easy for me as a person with 3 years of mechanical engineering under my belt to understand when broken down into steps

2 - ((All Good)): Relatively easy for me to understand

1 - ((Easy as Pie)): Easy as Pie!

So this is all based around my experience and there are probably geniuses who will find all of it easy and there will also be others who don't have a big background in math or science who might find it harder. If you spend the time thinking about it critically though, you will come to understand it which is the really cool thing about math and physics!!

Exponential Functions ((2))

So here's a nifty trick, if you're ever out and about and have forgotten your phone or calculator and really really really need to know what the exponential function of some number is (e^x) all you have to do is use the algorithm 

x = some number for examble 2!

e = the exponential function

k = a number which starts at zero and increases in jumps of 1 each additional term

! = means factorial. When a number has a factorial sign beside it you write down all the whole numbers from 1 to your number and put multiplication signs in between i.e. 4! = 1x2x3x4 = 24!!

So the equation says to begin with k = 1 and go to k = infinity but you don't need to do that. Noone does that because that's a really lame thing to do with you life and you're always going to fail. You just need to go until the equation converges. And by converging I mean when you are adding extra terms to the equation and it doesn't seem to be making much difference. Give it a go with x = 1. 

See if you can "converge" to 2.7 :D go on !

Here's just one example of where it might come in handy...

Matrix Exponential Function ((3))

So if you want to give this a go but you're unclear about matrices I explain them here.You also might want to know more about matrix operations (multiplication, dot products, determinants etc) you can find them here  because it will take SOOO much time to explain them all. 

So we are basically just going to take the above equation for a linear equation and magic it to make it work for a matrix as well. The other thing we're going to do is add variation with time by putting in the scalar value t because that will make our equation really useful. A lot of applied math varies with time! 

So we want to work out e^(At) which is the exponential function of a matrix which varies with time. Here's the equation we have to use. See if you can spot the similarities to the equation above...

So when we are expanding this out, instead using a 1 for the first term as we did in the above linear case we now use the Identity matrix [1,0  ; 0,1] for when k = 0 and . We simply expand as above to get our answer using a similar method as above. Let's do an example!! 

In this example A = [0,1;1,0]; We want to find e^At

using the equation above our terms are (just expanding out the equation):

= I + tA + (t^2)(A^2)/2 + (t^3)(A^3)/(2x3) +  (t^4)(A^4)/(2x3x4) ...

Subbing A into our matrix and using matrix formatting we get

Which seems really messy until we do this magical magical thing involving the series definition on this page. Now our matrix simplifies really beautifully to this...

Incidentally this is actually how your calculator calculates the sin and cos function. Think about it, it would be completely unreasonable to have manually tried to program in the sin and cos function. The calculator must be working it out somehow and this is exactly how! 

Modal Method - (the easy way)

So here's a wonderful application for the diagonalisation stuff we learnt about in one of my previous blogs! I love it when stuff is useful :D

We can calculate e^At in an easier way than the one above IF we know that matrix A is diagonalisable. 

BUT I'm going to save that for another night because my bed is calling and I still have a speech to write. 

GOODNIGHT EVERYONE!

<a href="http://www.bloglovin.com/blog/12644549/?claim=ar6ycwtrq4v">Follow my blog with Bloglovin</a>

Chitty Chitty Bang Bang and Business Plans

Why Failing at Business Plans Made Mr. Potts Fail at Life (well mostly)

Even with a really good idea, businesses can still be doomed to fail. Businesses don't want ideas, if they did, Mr Potts from Chitty Chitty Bang Bang would have been a billionaire from the get go.Businesses want money. Crazy right? And in order to do that, most of all, a business plan needs to be marketable. Let's be honest, Mr. Potts was an ideas man but not a realist. He got lucky with the flying car but the other stuff, when put onto a market, is worthless.

Mr Potts Doing His Thang

Take this breakfast making contraption for example https://www.youtube.com/watch?v=RBJGpNTP_lY. While pretty much one of the coolest things you will ever see in your life, its not going to catch on. This is because he didn't follow all the basic steps for making a business plan...

1. He didn't start with a problem: If he had gone out and interviewed people who cooked in their own home, they might have been able to tell them that they had no problem cooking simple foods like eggs and sausages in their own home. What they actually wanted was for their food to stop sticking to their fry pans. He also might have discovered that people generally wanted more space in their kitchen and did not want it to be taken up by a bulky, intricate contraption which is only able to cook two types of food! 
2. He didn't Identify Customers: The customer for this product are people who really really really like eggs and sausages, all the time for every meal and can't be bothered spending the time making them. This is an almost nonexistent market (pretty much just the Pott household) and should have been assessed before even beginning to make it.
3. He didn't Justify Competitive Value: This isn't as relevant to Mr. Potts because he failed to address a problem and a customer in the first place. But, this step is really important and involves thinking about what you could offer which would make people choose your product over existing products. 
4.  Ideas: To be honest he doesn't have a problem with ideas. He has one beautiful mind. Dat lateral thinking.
5. I guess he kind of thought about distribution: I'm going to use another example for this because it's my blog and I can do what I want to. I want to talk about the  toot sweets. Great idea to bring the toot sweets to a candy factory. Instant loyal customer base right there. 
6. Revenue: Where does the money come from, how much is needed and when? Be specific Mr. Potts, you're  never going to get anywhere in life if you don't think of all this stuff.
7. Costs: Not once in the interview with the sweet factory owner did he mention price. And the boss blindly accepted this. Some day someone's business is going under...
8. Resources: Did you know that if Mr Potts had a partner that businesses would be much much much more likely to invest in his ideas? If you're alone in thinking an idea is good, investors will question why that is...

You Forgot to do Market Research on any of your Products Mr Potts!!

Once you do have an idea, its important to validate it. Ask around and then answer these questions...

can you find customers?

do they have this problem?

is it important?

will they pay for it?

what are the key features of that solution?

Of course you should never people directly if they will buy your product because they will most likely say yes. People like saying yes. But in reality people are lazy and if they don't have a major problem with something, they won't change it. This is why its so so important to do your research! 

Mr Potts Should have Tried a Lean Set Up 
This is all about doing as little as possible to get the maximum possible output in order to attract in investors attention. Investors do not need to see finished prototypes Mr Potts! All they want to see is that the idea has potential and low risk! Ventures don't usually fail because the invention doesn't work, they fail more often because people don't want to buy it. 

WISE UP MR. POTTS!

Clothes Scrambles, Polymer Stiffness and Why Size Does Matter if You're Plastic

My Fourth Blog! Not bad...

Clothes Scramble! 

Second hand shops are great. Clothes scrambles are pure genius! Thank god for impulse buys and changing tastes! I had forgotten that there was one on today and when I figured it out I was so happy. My little brother just happened to snap a picture of my face at that moment of joy...

This clothes scramble had a small donation fee for two very good causes (SPCA and Sweet Louise). And yay! Clothing!

Polymer Necklaces

So I want to have a look at what gives a polymer different properties and not just whether it is a Thermoset or a Thermoplastic. If you do want to review that and what a polymer and a Mer are you can view my previous blog post here http://math-machines-and-playing-pretend.blogspot.co.nz/2014/07/measure-for-measure-thermosets.html

Remember last time we compared polymer strings to beaded necklaces? Well we're going to use that analogy and develop it a little further to have a look at the properties of a chunk of polymer.

Here's three terms to add to your vocabulary of smart sounding stuff:

Intramer Structure: This is the structure of the Mer itself. This refers to how the Mer structure is made up of chemical elements. Think of this as what the bead is made out of and how it is designed to be connected to the necklace.
Intramollecular Structure: (notice the "a") This is the way Mers combine to form polymer chains. If you want you can think of this like the string or the links between the beads.
Intermollecular Structure: How the chains interact with each other. Remember how previously we talked about Van Der Wal and covalent bonds and related them to tangles in the beads strings and perhaps a splash of superglue.

A really really important property of a polymer is its flexibility or rigidity. Take a look around you right now and find a plastic thing. Now ask yourself how absolutely useless this thing would be if that thing had different flexibility properties. The thing closest to me is one of those dog ball thrower things. Although not disastrous without it, a little bit of flexibility is good for this as it provides a bit of spring when throwing a ball. Even though it's a pretty trivial object, someone would have thought this through and intentionally chosen a polymer to balance price and stiffness.

When we say that a plastic is flexible what we literally mean is that its polymer chains can be easily slid over each other without causing permanent damage to the structure of the object. The three structure types that were introduced enough each have an effect on the flexibility. The Intramer structure has the greatest effect and the Intermollecular structure has the smallest effect. Let's examine them one by one...

Intramer structure: This is mostly determined by the atoms that make up the Mers. All atoms within molecules are joined to the other atoms by strong covalent bonds. Depending on the type of atom there can be single bonds or double bonds (in other cases I'm pretty sure there can be more as well). Bulky side groups can also form, an example of this can be seen below. Along with double bonds they are fantastic at making a polymer more rigid. If you're having trouble picturing how those two things can limit a polymers flexibility, Imagine trying to slide a rigid and spiky surface across another rigid and spiky surface. Yeah.

Intramollecular Structure: Different types of plastics form different lengths of polymer chains. And in this case, size matters. Imagine getting a whole bunch of necklace strings as big as your little finger and stirring them around in a bucket. Now Imagine getting another bucket, filling it with necklace strings a meter long and giving that a super long stir. Which bucket of necklaces is going to be the easiest to detangle? It's clear to see that when the average length of the polymer string goes up (and hence the molecular weight) the stiffness of the material will also increase.

Intermollecular Structure: It's all about the Van Der Wal bonds!! When in liquid form, the polymer strings are able to move around like wriggling worms. By really really quickly cooling the polymer down (or quenching it) you can freeze the wriggling worms mid wriggle. But if you cool the polymer down slowly then the worms will line up to form a crystalline structure which looks a little bit like zebra stripes. Take a look at the difference between these two structures...

Look how much more dense the polymer strings in the crystalline structure are! Because of this density the Van Der Waal bonds (which act between two polymer strings) will have more of an effect, they're a little bit like magnets because they pull more strongly when they are closer to another polymer string. And hence the material is also made stiffer by allowing the plastic to slow cool!!

Crucible Badges, Eigenvalues, Eigenvectors, Diagonalisation and Pretty Hair

Mages in Space! (who do their hair nicely)

So its pretty clear for those of you who have spent enough time with me, that I adore being a girl. Mostly because makeup and clothing are some of the coolest things on the planet and it's more socially acceptable for girls to enjoy them (but let's not open up that can of worms right now). I'm unashamed to say that this is one of the biggest reasons that I love things such as Larping and Theatre. All the dressing up!!

Today I am going to a mages in space larp and I have excitedly done my hair differently. Its pretty simple but I like it looots :D I french platted it back (always a struggle because I am way better at inverse braids) and then created a braid bun and put the flower in the middle of it all. Uber cool and took me 3 minutes to do.

To complete with my outfit I have brought my dirty overalls in the hopes of being some snobby first ship engineer. Its kind of gross that they are still grubby with car grease but it certainly adds to the outfit. 

Crucible Badges!

So special snowflakes and Darkest Past players better watch out because I have the following badges! Brace yourselves! 

Eigenvectors and Eigenvalues - The Sliced Bread of Mathematics 

Eigenvectors and Eigenvalues are simply a property of any matrix which some clever cookie discovered were very useful in real life applications. You may not know them yet but these bad boys have improved your life. They were used to design the suspension springs on your car, they could have been used to design the foundations of the building you are sitting in, they even helped you out that one time on Facebook where you posted some photos and Facebook correctly guessed which of your friends were in the picture. And best of all, they're pretty simple to calculate. I'm introducing them now for your benefit because I will probably be talking about them later on. I'll explain them then I encourage you to give my example a try! 

The Matrix (the real deal not the movie)

For those of you who aren't familiar with matrices (the rest of you can skip to the next paragraph) they are basically just a part of a set of linear equations. In this case because there are two rows, there are two equations which might be -5a + 2b = 4 and 2a -2b = 5. Don't panic! All I've done is taken the first half of both the equations, stacked them on top of each other and then factorized out the a's and b's. (I'll show you visual learners that in a picture). Also from now on (because its easier I will be representing matrices using the following notation [-5, 2; 2, -2] because its easier. ; indicates that the following numbers are on a lower row and , indicates that the following number is on a different column)

Eigenvalues 

Alrightie, the first thing you have to do is recognize that the definition of an eigenvalue is det( I lambda - A) = 0 where lambda is a vector of your eigenvalues. I know that seems super duper weird and complex but I'll break it down for you.

"det" stands for the determinant of a matrix. Its just another useful property so don't freak out! A 2x2 matrix e.g [1, 2; 3, 4] can be found by multiplying the top right and bottom left elements of the matrix and then subtracting the top right element timed by the bottom left element. So for the example matrix I gave above this would be 1x4 - (2x3) = -2! Simple right. Good old primary multiplication and subtraction :)

"I" stands for an Identity matrix. This is one of the simplest matrices you will come across. Its just a matrix filled with zeros with 1's going down on a negative diagonal (\). for example a 2x2 identity matrix looks like this [1,0; 0,1].

"lambda" is just the two eigenvalues you want to find. Lambda is the name of a fancy Greek symbol but blogger doesn't know how to speak Greek so I can't write it in text but I can give you this link to good old wiki which has a picture of it http://en.wikipedia.org/wiki/Lambda

Lastly A is just the matrix that you are trying to find eigenvalues and eigenvectors for. Which in this case is our old friend [-5, 2; 2, -2].

So putting this together we have the following working...

Eigenvectors

Cool, cool cool cool. Now we have our eigenvalues we can use them to get the corresponding eigenvectors. Let's start with our eigenvalue -6.

• The formula to get your eigenvectors is AX = lambdaX. Once again A is your matrix, lambda is a single eigenvalue. X represents your eigenvector that you are going to work out which for a 2x2 matrix looks like [x1 ; x2]. 
• We expand out our equation to get 2 linear equations (can be seen in the working below this massive chunk of text).
  Now I know what you're thinking, by now you will already have tried to solve the two equations for x1 and x2 with no success right :P ?? If you have 2 unknowns and 2 equations you can solve something right?? WRONG! well, its right but in this case it doesn't apply. This is because of the way you worked out your eigenvalues using the A matrix. These two equations are actually the same equation with a couple of things thrown in. So if you try to solve it, the answer will always be zero. You need 2 distinct equations to solve something with 2 unknowns. But never fear the next step will explain all.
• Eigenvectors are special because they don't just have one solution. They are able to have any solution so long as the ratio of X1:X2 remains the same. All we have to do is guess a value for X1 or X2 and using only one equation, work out the other X with respect to that value. To make things easy I am going to guess that X1 =1. 

Give the other eigenvector a go using lambda = -1. See if you can get some scaled version of [1;2] :) YOU CAN DO IT!

Test Yourself! 

Try this matrix out for size [0, 1; -2, -3]. See if you can get to the eigenvalues -1 and -2. Then see if you can get some scaled version of the eigenvectors [1,-1] and [1,-2]. If you do it I will give you a hi five next time I see you.

Diagonalisation Time

So like many concepts in math, diagonalisation has a really complex looking definition. Luckily some smart cookies have come along and said "Hey, do you know what would make this a lot easier? Just *insert brilliant world changing idea here*". 

A matrix A can be diagonalisable if you can find a matrix which is able to be used to transform your matrix into a matrix of the same size where the only non zero values are the ones on the diagonal *takes deep breath*. Mathematically this looks like this:  

Luckily there are a number of very clever people who have figured out that...

• If the matrix A (having dimensions nxn) is diagonalisable, then there are n linearly independent eigenvectors of matrix A.
  In other words if you have a 2x2 matrix, if you can prove that there are 2 linearly independent eigenvectors then you have proved that the matrix is diagonalisable. 

This is AMAZINGLY AWESOME!! because we already know how to figure out both eigenvectors and whether two vectors are linearly independent. here is a link to my previous blog where I talk about linear independence 

Tidy Rooms, Thermosets, Thermoplastics Necklaces for Saturday's Party!

Good Afternoon Beautiful People!

Post number two of my blog and hopefully on the way to a habit. I really appreciated those of you who had a keen enough eye to tell me where I'd slipped up. You guys are awesome because as I said I am using these notes as a study resource so you may have saved my future self some confusion as I revise for all my exams at the end of the year. I hope people learned some stuff (or revised some stuff for all of you smarties who already know all about linear independence). Onto post number two...

Apparently My Carpet is Cream Coloured

Today I got my act together and tidied my room! Dad, if you ever read this blog here is photographic evidence that my floor still exists.

I love tidying my room almost as much as I love game of thrones (and I love game of thrones a lot!). These two passions of mine are slightly connected by the fact that I found game of thrones audio books on youtube... Speaking of game of thrones the coolest cool cat of all is coming over to watch season 3 with me tonight. Tessa, prepare yourself for the continuation of George RR Martin's soul crushing plot! 

Thermoset and Thermoplastic Polymer Challenge

So we're going to do a bit of a murder mystery minus the murder. Most of this is a bit of revision for me because it was pretty much all in my chemmat 101 class. But its still super fun to go over and relevant to my 747 paper. Below is a picture of me holding up two types of pastics  (and no I do not think metal is a plastic, I'm talking about the handle of the steamer pot). One of them is a thermoset and the other is a thermoplastic. See if you can guess which is which when I've finished explaining why they are different at a molecular level. Good luck Sherlock! 

Firstly, I wanted to introduce you to the concept of Mers (something most chem heads will know about). Mers are the building blocks of polymers. They are simply a  repeating chemical structures which are able to link together to form long chains. Think of them like beads on necklaces if you want. Then imagine that your chunk of polymer is a pile of beaded necklaces. And depending on how long the necklaces are and whether the beads are covered in Velcro you will get different properties from this pile of beads. You may be able to add a little bit of force to tear them back apart or else the pile may be so tangled and stuck that trying to separate them will only destroy all of your precious jewelry. Keep this in your head. You're doing awesome :)

Thermosets are made by pouring Resin (which is maybe a sort of plant secretion) into a mold with a catalyst, mixing it all up with maybe a little bit of heat and leaving it to cure or set! At a molecular level this results in a network of criss crossing polymers. A chemical reaction was what caused the plastic to set so there are strong covalent bonds or cross links joining the strings of polymers. You can never reverse this process. And if you try to heat the polymer up its only going to burn away leaving only a wonderful smell and some carbon behind. Imagine taking all your beads and putting them into a bucket and then squirting superglue into the bucket, swishing all the beads around and leaving it to dry. Are you ever going to reclaim your favorite string of beads for the party on Saturday? ONLY IF YOU DESTROY ALL YOUR NECKLACES IN THE PROCESS! 

Thermoplastics have got their own thing going on. Things made out of thermoplastic are formed by taking some itty bitty plastic pellets or solid stock, adding some heat and rolling them out and reshaping to get whatever you want. The strings of polymers are connected through secondary bonds or Van Der Wal bonds. These are much much weaker than covalent bonds and can be broken with a little bit of heat. This means that thermoplastics can be processed over and over and over again as long as you don't dump them in landfill. Lets look at your necklace pile again. This time you've stuck them in a bucket without super glue and given them a stir. Even though they will get mega tangled, you're still able to extract them using a little bit of time and finger power and wear them to the Party on Saturday. 

So have you got it? The milk bottle is recyclable and not as rigid as the handle. Also the handle is used in a high heat application making it a little inappropriate if it changed shape every time you steamed some veges. Bottle = Thermoplastic and handle = Thermoset!  Yay!

More on these later! But for now I have a lecture to go to and two classes to teach then GOT to watch :)

A Hello from Me, Linear Systems and Measure for Measure

Introductions

HELLO! THIS IS MY BLOG!

HERE IS A PHOTO OF ME LOOKING HAPPY WITH MYSELF FOR CREATING THIS BLOG!

I've tried making blogs before and blogged about my thoughts and crazy dreams but I thought that I would do something different. So here are my experiences as an amateur actress, a LARPer and a mechanical engineering student. I'm going to be using this as a bit of a study resource as well as a thought map so if you don't like learning things, best not read the sections with titles like "algebra rules for multivariable control systems" or "thermoplastics processing". So i'll get on with it :D

Another Play!

FANTASTIC news today! I've been shortlisted for a part in Measure for Measure (a play from the wonderful Shakespeare) at shoreside theatre. Recalls are next Tuesday and needless to say I am very very excited. The last time I was in a play was last year in December as a pantomime villain Dan Dini at Mairangi Players (picture top right) and the last time I was in the Shoreside Summer Shakespeare was when I was 15. I played William the simpleton in As You Like It (picture bottom right).

Measure for measure is actually a really interesting play about morality and different views on *cough* fornication. I'm currently making my way through the play with reference to the English interpretation (because Shakespeare is totally a foreign language right? :P ). Here's the link to the text with modern interpretation http://books.google.co.nz/books?id=Vg8wXH-2kvoC&printsec=frontcover#v=onepage&q&f=false

Jordan and Amy and Mark and Jackie have also been called back so needless to say I'm really really excited for Tuesday and the rest of the play!!

Linear Independence

Firstly, here's an introduction to matrices if you have no prior knowledge but really want to challenge yourself by reading on. http://www.purplemath.com/modules/matrices.htm

Basic Concept 

So another exciting thing that happened today is that I understand linear independence! Linear independence is a relationship between vectors (in control systems, these vectors are mostly within matrices). The basic concept is that if two vectors are linearly dependent they can be multiplied by a constant and summed together to cancel each other out. 

Visualization of Concept

A good way to visualize a vector is as a coordinate or an arrow. Here's a picture I drew for you!

Linearly Dependent Arrows

The red and the orange arrow are linearly dependent. Visually we can see this as they are both on the same straight line with the same absolute gradient (somewhat limited by my drawing skills in paint but you get the point). The orange vector can be multiplied by a constant of approximately 2 and added to the red vector to cancel out completely. A mathematical example of linear dependent vectors is [2,4] and [4,8] because 2*[2,4] + -1*[4,8] = [0,0]

Linearly Independent Arrows

My second drawing is of linearly independent vectors. They have different gradients so no matter how much you make the arrows grow or shrink (mathematically speaking, no matter what constants you multiply them by) they will never be able to be summed in order to cancel each other out. A mathematical example of linear independent vectors is [2,4] and [3,1]. the ratio of the first and second numbers is different in each of the vectors so it is impossible to times them by a constant in order for them to cancel each other out. No, you are not allowed to multiply them by zero in order to cancel them you sneaky sneaky thing. Also vectors do not count as constants so don't even think about multiplying one of the vectors by another vector.

Rank Limitations and Magic

The magical thing about vectors (and this is really awesome) is that when you have a matrix, you can never ever ever ever have a matrix with more linearly independent vectors than the minimum dimension of the matrix.

For example: if you have a 4x2 matrix [3,2;  4,5;  6,2:  3,1] you can never have more than 2 linearly independent vectors. Any conceivable vector can be created by forming a linear equation using one or more of the linearly independent vectors multiplied by constants. e.g [3,2] = a[4,5] + b[6,2]. We know it is possible to find out a and b because there are two constants and two unknowns!!! MAGICAL RIGHT??

Determining Rank (Full Rank?)

Determinant Method of Determining Rank

There are two nifty ways to figure out if a matrix is full rank. The first method is to find the determinant of the matrix and if it is not equal to zero, the matrix is full rank (det(A) /= 0 ---> A is full rank) This is best used on equations with an equal number of rows and columns. You can use it on 2xn matrices by going through and testing each column against the other columns for linear dependence. This is illustrated to the left. All combinations of vectors need not be tested. Some vectors can have an implied linear dependence. For example if the vectors in the green square have a determinant = 0 and the vectors in the blue square have a determinant = 0, it is implied that vector [a,b] is linearly dependent to vector [d,e] even though this vector combination was not formally tested. Cool right? 

(or not)

The second method of testing is more suited to matrices with unknowns and such or long 2xm matrices if you can't be bothered finding out ALL the determinants. You can simply look at individual vectors, and figure out if they can be multiplied by a constant to create another set of vectors within the matrix (this is only for the case where a vector is dependent on only one linear dependent vector but in trickier cases a vector can be dependent on two or more). If this is possible then only one of the vectors is counted as linearly independent. All the other vectors are clones if you will. They may have different hair cuts and clothing but each has the same DNA. By using this methodology and using implied dependence techniques a solution can be very quickly reached. Here is an example of a problem suited to this second method of doing things:

Topic For Business Plan 

In 703 we have to find an idea for an innovation to market and I have decided to do something a bit larpy. I want to make a large scale GM app for WOD games. Something that will hopefully make large scale combats a lot quicker and smoother to add to the immersion of the game. It allows players to be alerted when their initiative is reached and sends the success of their rolls directly to the GMs. It can be downloaded onto smart phones and bought from the WOD creators for a small fee. The plan is that it will not only make a profit (about 50c per download) but it will also help grow the game which is somewhat limited by how awkward combats can be. I'm pretty excited about this idea so LARPers watch out! I will be doing market research on you!!! :D

Goodbye!

I doubt anyone will read to the end but congrats if you have! If I am good I will post again tomorrow. Otherwise you may abuse me for clearly not doing my homework! CYA!